Sec 3 Theta 2 2
The secant office 'or' Sec Theta is 1 of the trigonometric functions apart from sine, cosine, tangent, cosecant, and cotangent. In right-angled trigonometry, the secant function is divers as the ratio of the hypotenuse and adjacent side.
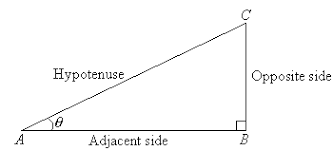
The mathematical denotation of the secant function is,
\(\sec(\theta) = \frac{\text{Hypotenuse Side}}{\text{Side by side Side}}\)
More About Sec Theta
The derivative of \(\sec(\theta)\) in calculus is \(\sec(\theta) \tan(\theta)\) and the integral of it is \(\ln|\sec(\theta) + \tan(\theta)|\). The reciprocal of \(\sec(\theta)\) is \(\cos(\theta)\).
Below is a tabular array of sec theta values for dissimilar degrees and radians.
| Radians | Degree | Tangent Value |
| 0 | 0° | one |
| \(\frac{\pi}{vi}\) | thirty° | \(\frac{2}{\sqrt{3}}\) |
| \(\frac{\pi}{iv}\) | 45° | \(\sqrt{two}\) |
| \(\frac{\pi}{3}\) | 60° | two |
| \(\frac{\pi}{two}\) | 90° | \(\infty\) |
| \(\pi\) | 180° | -1 |
| \(\frac{3\pi}{2}\) | 270° | \(\infty\) |
| \(2\pi\) | 360° | one |
Important Sec Theta Formula
Some important properties of the secant part and sec theta formula are:
- \(\sec(-x) = \sec(10)\)
- \(\sec(90° – x) = cosec(ten)\)
- \(\sec(x + two\pi) = \sec(x)\)
- \(\sec(\pi – x) = -\sec(x)\)
- \(\sec^ii(x) = i + \tan^2(x)\)
- \(sec(ten + y) = \frac{\sec(x) \sec(y)}{one – \tan(ten) \tan(y)}\)
Solved Examples
Question one. If \(\cos(x) = \frac{3}{five}\), calculate the value of \(\sec(x)\).
Solution. Using trigonometric identity,
\(\sec(x) = \frac{ane}{\cos(x)} = \frac{one}{iii/5}\)
\(∴ \sec(ten) = \frac{5}{3}\)
Question two. If \(\tan(\frac{x}{ii}) = \frac{v}{8}\), calculate the value of \(\sec(ten)\).
Solution. Using trigonometric identity,
\(
\begin{align}
\sec^ii(x) & = 1 + \tan^2(x)\\
& = ane + (\frac{5}{viii})^2\\
& = \frac{89}{64}\\
\sec(ten) & = \frac{\sqrt{89}}{8}\\
\end{align}
\)
Question 3. Prove \(\sec(10 + y) = \frac{\sec(x) \sec(y)}{i – \tan(x) \tan(y)}\)
Solution. As we know,
\(\sec(x) = \frac{1}{\cos(ten)}\) and \(\tan(ten) = \frac{\sin(x)}{\cos(x)}\)
Considering RHS of given equation,
\(
\brainstorm{align}
\frac{\sec(ten) \sec(y)}{1 – \tan(x) \tan(y)} & = \frac{\frac{1}{\cos(x)} \times \frac{ane}{\cos(y)}}{i -\frac{\sin(10)}{\cos(x)} \times \frac{\sin(ten)}{\cos(y)}}\\
& = \frac{ane}{\cos(x) \cos(y) – \sin(10) \sin(y)}\\
& = \frac{1}{\cos(x + y)}\\
& = \sec(x + y)\\
\finish{align}
\)
Hence, LHS = RHS … Proved
FAQs
Explicate how sec(-x) = sec(x).
As we know, the angle (-x) lies in the 4th quadrant of a graph, and the secant is positive in this quadrant. Hence, sec(-x) = sec(x).
What is sec theta?
Sec theta of an angle in a correct-angled triangle is defined every bit the ratio of the hypotenuse and next side.
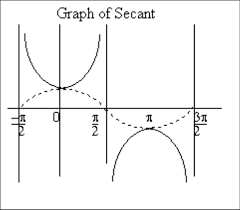
In which quadrants is the secant role positive and in which quadrants is it negative?
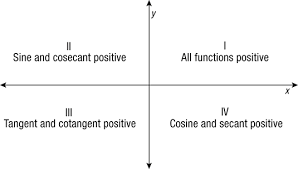
It can be observed from the higher up graph that sec(x) is positive in the 1st and quaternary quadrants and negative in the 2nd and tertiary quadrants.
Know More Trigonometric Function
Sec 3 Theta 2 2,
Source: https://protonstalk.com/trigonometry/sec-theta/
Posted by: warrenwifichaved.blogspot.com


0 Response to "Sec 3 Theta 2 2"
Post a Comment